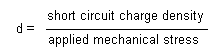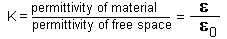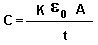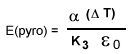CHAPTERS
CHAPTER 1
Terminology
- Introduction
- Relationships
- "d" Constant
- "g" Constant
- Dielectric Constants
- Capacitance
- Coupling Coefficients
- Young's Modulus
- Density
- Dissipation Factor
- Mechanical (Qm)
- Curie Temperature
- Aging Rate
- Pyroelectricity
- Performance
CHAPTER 2
Glossary
| Bimorph | A common term for "bender" or flexure element. Two layers of piezoceramic with or without a center shim. |
| Capacitance (free) | Referring to capacitance measurement on piezoelectric sample, the capacitance value obtained with sample being totally free of mechanical constraint during measurement process. |
| Capacitance (clamped) | Referring to capacitance measurement on a piezoelectric sample, the capacitance value obtained with sample being totally constrained from motion (i.e., "clamped") during measurement. |
| Coercive Field | Relating to a uniform ferroelectric material, the electric field required to oppose and bring to zero the internal polarization. |
| Charge Constant (d) | see "d" Constant |
| Compliance (s) | see "s" Constant |
| Coupling Coefficient (k) |
A dimensionless number related to the effectiveness of electrical to mechanical energy conversion in nonresonant piezoelectric devices. |
| Curie Temperature: | Temperature at which the crystal structure undergoes a phase change from non-symmetrical lattice (such as tetragonal) to symmetrical lattice (such as cubic). Drastic dielectric and piezoelectric coefficient changes accompany this phase change. |
| "d" Constant | The piezoelectric constant relating applied electric field to resultant strain (units of meters per volt). |
| Density | In reference to piezoceramics, the mass per unit volume of a fired ceramic body. High density is an important measure of the quality and uniformity of ceramic material because it indicates the absence of microscopic pores which weaken the ceramic and cause poling difficulties. |
| Dielectric Constant | see "K" Constant (capital "K"!) |
| Electrostrictive Materials | An important class of solid state motor materials which exhibit strains proportional to the square of the applied electric field strength. (By contrast piezoelectric materials exhibit strains directly proportional to the applied field strength. |
| Ferroelectric Materials | Class of crystalline materials which possess an internal polarization which can be reversed by an externally applied field, i.e., a crystalline material which can be poled. All of the commonly used piezoceramics are ferroelectric. |
| Flaw Detection | An early practical application of piezoelectrics, taking advantage of the fact that ultrasonic sound waves travel freely in a solid object (such as a steel girder) until they hit an air bubble or a structural flaw, at which point they reflect backwards and are detectable. |
| Frequency Constant | see "N" Frequency Constant. |
| "g" Constant | Piezoelectric coefficient relating the stress applied to a crystal to the resultant electric field in the crystal (units of volt meters per Newton). |
| "K" Constant (Dielectric Constant) | For a piezoceramic material, the ratio of its dielectric permittivity to the dielectric permittivity of free space (8.85 E -12 Farads per Meter). |
| Mechanical Q | Referring to both piezoelectric materials and non-piezoelectric materials - the INVERSE of the percentage of mechanical input energy which is converted to heat (the balance being stored as recoverable elastic energy). A material with Q = 100, when subjected to 1.00 Joules of work, will lose .01 Joules to internal heating. |
| "N" Frequency Constant | Referring to resonating piezoelectric bodies of various geometries - the product of the resonant frequency and the length of the body along the axis of motion for the designated vibrational mode. |
| Piezo Electricity | An observed phenomenon in some crystals - the generation of surface charges in response to applied stresses. |
| Pyroelectricity | An observed phenomenon in some crystals - the generation of surface charges in response to the application of uniform temperature changes. |
| "s" Constant (compliance) | In an elastic body, the ratio of uniaxial strain to uniaxial stress. |
| Saw Device | Piezoelectric acoustic device employing surface acoustic waves. |
| Sonar | Name given to piezoelectric application consisting of measuring distances to submerged objects by broadcasting a sound "pulse" and timing the reflected signal. |
| Strain | The ratio of change-in-length to length. Used in describing the effects of applied stresses or applied electric fields. |
| Stress | In a mechanical sample, the ratio of applied force to cross-sectional area which bears that force. |
| Ultrasonic | Refers to the frequency of operation of an electric or acoustic device - a general term for "higher than 20 kiloherz". |
| Voltage Constant (g) | see "g" constant. |
| Young's Modulus | Elastic modulus of a solid sample, i.e., the ratio of stress in a sample to applied strain. For practical purposes, Young's modulus is equal to the inverse of the appropriate "s" constant. |
CHAPTER 3
List of Symbols
| Symbol | Name | Unit |
|---|---|---|
| A | Area | m2 |
| C | Capacitance | F |
| D | Diameter | m |
| Di * | Dielectric displacement | C / m2 |
| dij * | Piezoelectric charge constants | C / N |
| Ei * | Electric field components | V / m |
| Ec | Coercive field | V / m |
| Fr | Resonant frequency | Hz |
| F | Force | N |
| Fb | Blocking force | N |
| gij * | Piezoelectric voltage constants | V m / N |
| G | Shear modulus | N / m2 |
| k | Electromechanical coupling coefficient | |
| k33 | Longitudinal coupling coefficient | |
| k31 | Transverse coupling coefficient | |
| k15 | Shear coupling coefficient | |
| kp | Planar coupling coefficient | |
| kt | Thickness coupling coefficient | |
| keff | Effective coupling coefficient | |
| KijS * | Relative dielectric constant at constant strain | |
| KijT * | Relative dielectric constant at constant stress | |
| L | Length | m |
| p | Pressure | N / m2 |
| ρ | Pyroelectric coefficient | C / m2 K |
| Pi | Polarization components | C / m2 |
| P | Power | W |
| Q | Mechanical quality factor | |
| Q | Electric charge | C |
| Qs | Short circuit charge | C |
| R | Electrical resistance | Ω |
| sijE * | Elastic compliance at constant E | m2 / N |
| Si | Strain components | |
| Smax | Maximum recommended strain | |
| t | Time | s |
| t | Thickness | m |
| tr | Response time | s |
| T | Thickness | m |
| Ti * | Stress components | N / m2 |
| V | Volume | m3 |
| V | Electrical voltage | V |
| Vo | Open circuit voltage | V |
| vs | Velocity of sound | m / s |
| x | Deflection | m |
| xo | Free deflection | m |
| α | Thermal expansion coefficient | 1 / K |
| εo | Dielectric constant of free space | F / m |
| ρ | Density | kg / m3 |
| ρ | Electrical bulk resistivity | Ω m |
* j = 1 to 6 |
||